SPLITT traversal modes

Venelin Mitov

Prune ranges

To maximize the potential for parallel execution SPLITT divides the node-states during a tree traversal into a InitNode, a VisitNode, and a PruneNode operation (see the Writing a travefsal specification guide for a description). Further, SPLITT rearranges the node-ids (and, hence the data) associated with the nodes in the computer memory, so that the InitNode and the VisitNode operations can be performed on ranges of consecutive node-ids. Performing these operations on a range of consecutive addresses in the memory has a potential to increase the efficiency of computation, because modern CPUs provide vectorized instruction sets, i.e. low-level processor instructions, such as (addition and multiplication) that can be executed simultaneously on a group of 2, 4 or more consecutive words in the memory. Another benefit from ordering the operations over such ranges is that the data associated with these nodes will tend to be less fragmented and, therefore, it will be found more often in the processor cache.

To orchstrate this process SPLITT divides the traversal in a number of phases. The diagram below is an example in which the traversal of a tree is divided into an Initialization phase and four consecutive phases called “prune-ranges”:

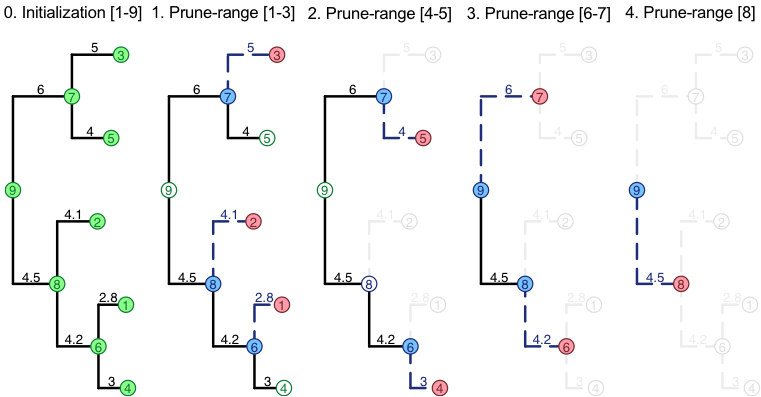
In each phase, full nodes in green denote parallelizable executed InitNode-operations, full nodes in red denote parallelizable VisitNode operations and full nodes in blue denote parallelizable PruneNode operations.

In general, there is no arrangement of the nodes in the memory, in which both, the VisitNode and the PruneNode operations will be executed on ranges of consecutive nodes. For example, the range of daughter-nodes 1, 2, 3 corresponds to the parent nodes 6, 8 and 7. In a bigger tree, the parents could well be nodes 6, 25, 12 or any other non-consecutive ids. Thus, the efficiency of the calculation can, in principle, be affected by the order in which the ranges of VisitNode- and PruneNode-operations are processed. SPLITT provides several possible arrangements (called “orders” or “parallel models”) of the execution cycle of these operations, which are all equivalent with respect to the result of the calculation, but may have different parallel efficiency, depending on the tree and the application-specific traversal operations.

Parallel traversal modes during post-order tree traversal

• AUTO 0
• SINGLE_THREAD_LOOP_POSTORDER 10
• SINGLE_THREAD_LOOP_PRUNES 11
• SINGLE_THREAD_LOOP_VISITS 12
• MULTI_THREAD_LOOP_PRUNES 21
• MULTI_THREAD_LOOP_VISITS 22
• MULTI_THREAD_LOOP_VISITS_THEN_LOOP_PRUNES 23
• MULTI_THREAD_VISIT_QUEUE 24
• MULTI_THREAD_LOOP_PRUNES_NO_EXCEPTION 25
• HYBRID_LOOP_PRUNES 31
• HYBRID_LOOP_VISITS 32
• HYBRID_LOOP_VISITS_THEN_LOOP_PRUNES 33